Answers: Trigonometry (degrees)
These are the answers to Questions: Trigonometry (degrees).
Please attempt the questions before reading these answers!
Q1
You are given the triangle below.
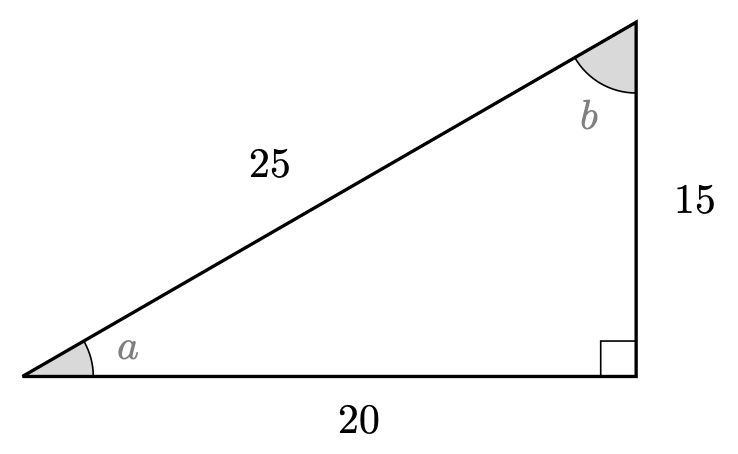
Here,
\(\cos(a) = \dfrac{4}{5}\)
\(\sin(a) = \dfrac{3}{5}\)
\(\tan(a) = \dfrac{3}{4}\)
\(\cos(b) = \dfrac{3}{5}\)
\(\sin(b) = \dfrac{4}{5}\)
\(\tan(b) = \dfrac{4}{3}\)
Q2
Using the triangle below, solve the following equations.

2.1. \(C = 12\)
2.2. \(A = 2\)
2.3. \(A = 1.812\) (to three decimal places)
2.4. \(A = \sqrt{6}\)
2.5. \(A = 8\)
2.6. \(B = \frac{8}{\sqrt{3}}\).
Q3
3.1. \(\cos(30) = \dfrac{\sqrt{3}}{2}\)
3.2. \(\tan(30) = \dfrac{1}{\sqrt{3}} = \dfrac{\sqrt{3}}{3}\)
3.3. \(\csc(45) = 1\)
3.4. \(\cot(30) - \sin(60) = \sqrt{3} - \dfrac{\sqrt{3}}{2} = \dfrac{\sqrt{3}}{2}\)
3.5. \(\sin(90) + \cos(180) = 1 + (-1) = 0\)
3.6. \(\tan(30) - \cot(30) = \frac{1}{\sqrt{3}} - \sqrt{3}\)
3.7. \(\cos(0)\sin(90) = 1\cdot 1 = 1\)
3.8. \(\cos(30)\sec(30) - \sin(45)\csc(45) = 1 - 1 = 0\)
3.9. \(\cot(90) = 0\)
Version history and licensing
v1.0: initial version created 08/23 by Dzhemma Ruseva, Ellie Gurini, Ciara Cormican as part of a University of St Andrews STEP project.
- v1.1: edited 05/24 by tdhc, and split into versions for both degrees and radians.